Lining

This next post was supposed was supposed to be on aligning timbers before performing the operations described in our initial look at the French scribe method. However, I have had the good fortune to be given a presentation on lining by Jean Pierre Bourcier, and I've decided to translate it from French and present it here. Lining is the preliminary step of marking reference lines on timbers. In particular, the reference lines are projections of an axis, used to align the timber on top of the full scale drawing, onto the faces of the timber. It works with irregular timbers and is a prerequisite to scribing. Without further ado, here is Jean Pierre's presentation, with a couple of my comments. If any reader spots phrases that could be better translated into specific timber framing terminology, please let me know in the comments.

Lining

Preliminary operation for using the niveau de dévers method with the ground plan.

Definitions

• Lining: tracing, on two adjacent faces of a irregular timber, the projections of a line chosen to be the timber's axis.
• Counter lining: projecting those lines onto the two opposing faces of the timber.

• Square line (trait carré): line perpendicular to a given line.
• pièce de niveau: timber that has been leveled along its length.
• pièce de dévers: timber that has been leveled across its width i.e., has no twist.
• Reference area (plumée): small rectangular area on a surface which has been dressed (planed) smooth, [ so that a level can be accurately placed on it]. This serves as a reference for lining and counter lining.

• to plumb: to trace a vertical line from a point by using a plumb line.

Step 1

Block up the timber so that its flattest face (abcd) is level lengthwise.

Step 2

Make a reference area in the middle of the face abcd at mnoq.

Step 3

From X at the middle of ab and X' at the middle of cd, draw the line XX'.

Step 4

Draw a perpendicular to XX' in the reference area.

Step 5

Turn the timber 90 degrees so that face abcd is vertical and face adef is horizontal.

Step 6

Make a reference area nqpr in the middle of face adef.

Step 7

With Y at the middle of the line af and Y' at the middle of line de, draw the line YY'

Step 8

Draw a perpendicular to YY' in the reference area that intersects point u. [u is the intersection of the perpendicular drawn in face adef's reference area with the edge of the timber.] This perpendicular intersects YY' at k'.

Step 9

Make line k'u level across the width of the timber. [The semi-circular object in the drawing is a plumb level.]

Step 10

• Choose a point i on the end of the timber. The plumb level is placed on this point. The vertical distance iX is transferred [across the face] to vw.
• [Draw Xw.]
• The reference is the horizontal edge of the plumb level, oo'.

Step 11

• At the other end of the timber, choose a point i1 and perform the same operation. The plumb level is placed on i1 and the vertical distance i1X is transferred to v1w1. The horizontal edge of the plumb level is still used as a reference.
• Draw line ww1.

Step 12

On the near face, put a plumb line against point Y. This defines point g on the line Xw and point t on the bottom face.

Step 13

• On the far face, put the plumb line against point Y' and mark point g' on the line X'w' and t' on the bottom face.
• Draw line tt' on the bottom face [(after turning the timber so you can do this operation)].

Step 14, the final result

• The four lines XX', YY', ww' and tt' represent the projections of the timber's axis onto the four faces.
• The axis passes through gg'.

Conclusion

The timber can now be aligned on the ground plan.

This procedure is directly applicable to assemblies in which the timbers' orientations are square to each other, like in roof trusses. In the previous post in the series on the French scribe method, we skipped directly to the "good stuff" of laying out timbers with twisted orientations. The lining procedure is still useful in that case too. Our example was the layout of a hip rafter and king post. The king post will also be layed out with rafters, so it will be lined in the manner described here. That establishes a central axis, seen here:

at the intersection of the green lines. The reference plane common to the hip rafter and king post will pass through that axis. Once we have the king post twisted to the correct orientation, we can use the plumb level to draw a level line through the central axis on both end faces of the timber. We then connect those to make the reference assembly lines that represent the reference plane.

Note: I found many translations of French carpentry terms at Marc Guilhemjouan's pages on traditional timber frame techniques.

Stereotomy Blog - the Making Of

For those who might be interested in how I do the illustrations for Stereotomy Blog, I've started a series on my computer graphics blog Shiny Dynamics. I use the open-source 3D modeling program Blender; my first post explains how to draw a convincing wood grain in Blender. Future posts will cover topics such as:

• Non-realistic shading used by technical illustrators;
  
• Emphasizing edges using the Freestyle rendering extension;
  
• Integrating geometry and images from a 2D CAD program;
  
• Drawing on objects using an external program, like in the images in the French scribe method post.
  

If your interests lie in that direction, check it out.

A Real Roof Model

Faithful reader Rob Simpson made a cardboard model of the roof used in the devers de pas series of posts:

Sometimes a physical model (and a nice glass of beer) are the best aids for understanding roof geometry!

Le Piquage, or Theory of French Scribing

We are going to start with some informal observations about the geometry of intersecting timbers and, using those, explain le piquage, or "French scribing" as it is known in the English-speaking world. This layout technique is much older than the rembarrement we looked at last time. There won't be any carpentry drawing today, but I find the technique fascinating, and we will soon tie it back to a drawing done at full-scale on the ground of the building site.

Here are the king post and a hip rafter from the model in the last entry:

The two timbers are not parallel, so we can take a long edge from each and displace them in space so that they intersect. Those intersecting lines define a plane. We can move the lines anywhere we want, so we can define an infinite family of planes that are all parallel to the edges of the timbers. Here are two such planes:

The plane on the right passes through the intersection of two roof surfaces on the hip rafter. We will use it as a reference to locate features on the two timbers. The left-hand plane is not very special; it is merely near the two timbers. But, its existence implies that any two timbers can be assembled against a plane. The planes and timbers can be tilted any way we like: for example, onto the ground, over a full-scale drawing of the layout:

(I've eliminated the carved top of the king post, which can be done later). This is a very useful fact for the carpenter. It means that any two timbers can be trial fitted on the ground, using a template, without needing to erect them into their final orientation in 3D space. The picture also presents the tantalizing prospect of being able to directly lay out the joint between the hip rafter and the king post, if only we could get them together into their final arrangement.

We can't do that before the joint is cut, because the timbers are solid. The closest we can do is move one of the timbers vertically, keeping the same orientation and longitudinal rotation. In other words, working backwards in time, we displace the hip rafter upward and fill in the joint:

On each timber we've drawn a line where our reference plane intersects them when they are assembled. If we choose a point on the hip rafter's reference line and move straight down to the level of the king post reference line, we will find a point on (or near) the king post that is coincident with the upper point when the timbers are joined together in their final arrangement. Also, we make a critical observation: each pairwise combination of faces in the hip rafter and king post have the same relative orientation in this configuration as they do when together, assuming everything is straight and flat. This implies that the intersection line between two displaced faces will have the same direction in space as they do in the final assembly, though the line will of course be in a different position. More formally, the direction of the intersection line is the vector cross product of the surface normals of the planes, and the normals are direction vectors without a fixed origin, so they are unaffected by the translation.

Let's see how we might lay out the joint, starting with the side of the hip rafter that is plumb i.e., the bottom in the final assembly. I am going to assume that the operations I describe can be performed accurately by a carpenter and save discussion of possible errors until the end. After the timbers are established in their correct relative orientation, the first step is to create a vertical reference line:

This is done with a special carpenter's plumb bob with a hollow center so that it can be aligned with lines on the ground. Looking down from above, the plumb line is aligned with the intersection of the hip rafter face and protruding arris on the king post. The vertical plane defined by the plumb line and rafter face is projected and drawn on the king post with the use of dividers.

The horizontal distance from the plumb line projection on the king post to the plumb line is measured:

That distance is transferred and marked on the hip rafter.

That point marks the location where the king post arris intersects the rafter's face in the final layout.

Next, we find the direction of the intersection line of the two faces by laying a straight edge against both faces:

We draw a line on the hip rafter, but it is obviously not in the correct position. We know that the true intersection line must go through the king post arris, so we draw a line parallel to the direction line that goes through the point we marked previously:

We now have one side of the joint marked out.

The intersection line on the king post is coincident with the perpendicular line in this case, because the rafter surface is plumb. In order to find where the second side of the joint intersects the first, we measure the distance from the king post reference line up to the arris along the intersection line:

And mark it on the rafter:

That point is also where the intersection line crosses the plumb line reference. That is no great surprise because the plumb line touches the corresponding point on the king post, but this will not be true in general.

Now we establish the intersection line direction for the upper face of the king post:

And move it to cross the line from the lower plane at the correct point:

To summarize, I've redrawn the complete layout on this side without the construction lines:

Now we turn our attention to the other side of the hip rafter. Here the situation is slightly more complicated because there are two faces to lay out on the hip, and they aren't plumb. Nevertheless, the principles are the same. Start by establishing the plumb line reference against the arrises of the two rafters:

Drawing the projection of the plumb reference plane on the king post will be a bit more artisanal because there is no vertical plane to use as a reference.

The common reference point is measured and marked:

The direction of the intersection line between the two lower faces is found:

and transferred to the known intersection point:

Next we find the intersection line between the lower king post and upper rafter faces:

At the same time we find the distance along this line between the horizontal reference plane and the upper king post face. On the other side of the joint we were able to measure distance along the actual intersection line on the king post; here we cannot. However, we are measuring the distance on a line parallel to the real line, so the distance will be the same. Assuming everything the faces are flat, of course.

The intersection line is moved to its correct position on the upper hip rafter face, and the distance we just measured is marked:

The final intersection, between the two upper faces, is found:

And transferred:

Voilà the joint layout on this side:

To finish up we connect the layouts from the two sides across the top face:

and the bottom:

The connecting lines are parallel to the king post edges, which confirms that we did the layout correctly.

This is the method that built the cathedral roofs of England and France in the Middle Ages. It is much more "concrete" than the rembarrement method we looked at last time: instead of dealing with abstract planes and using descriptive geometry to find their intersections, the actual timbers are directly used as a sort of graphical 3D calculator. The method relies on principles of geometry, but those do not have to be understood in order to apply it. On the other hand, quite a bit of skill would be required to carry out a real layout, and acquiring that skill was obviously a big part of the carpenter's apprenticeship.

This post is called "Theory of French Scribing" for a good reason. We have skipped many details of the process in order to concentrate on how the simple application of geometry leads to a layout method. The second case we encountered -- two timbers with no plumb faces -- is actually the most difficult to handle. It would be much more common to work with timbers with plumb faces, such as when laying out a principal rafter against the king post, or other parts of the frames. Here are some of the issues we blew off:

• We assumed that we can mark and measure horizontal and vertical lines in space by eye. This obviously takes a bit of practice. Today you can buy dividers with built in spirit levels, intended for scribing the joints in a log cabin. Medieval carpenters didn't have those. On the other hand, small errors doesn't necessarily have much of an effect on our measurements. When we measured horizontally from the king post reference line out to the plumb line, we would have to be 8 degrees out of horizontal in order to have a 1 percent error. If an apprentice's dividers were that askew, the master would surely throw something at him!
  
• We assumed that the timbers were straight and their faces flat. As I said the last time, one of the strengths of scribing is that it can handle warped timbers. An out-of-square timber is a special case of the general problem of laying out oblique faces that we just did, but in practice carpenters would use a faster method to deal with that. The intersection lines we found with the straight edge are usually close to the true intersections, so the results will be close, but judgment and skill are required.
  
• Any errors we did make could be expensive. In practice, some margin would be left and trimmed away in the final trial fit of the two timbers.
  
• Finally,this is personally theoretical because I've never done it! The pages of Mazerolle that explain the method are quite confusing. There is very little free information available on the Web on French scribing, and practically none explaining the method for oblique faces. I could be making a number of false assumptions.
  

Nevertheless, we now know enough about scribing to be able to understand the notation on carpentry drawings that are intended for that layout method. We said nothing about how the timbers are positioned before scribing. We will cover that next time and see how to go from the drawing -- done at full scale -- to the arrangement and relative rotations of the timbers. I teased you by displaying a plan under the timbers, so that should give you a hint about positioning the timbers in their common reference plane.

A Carpentry Model

We're back with a new look, courtesy of Blender and the Freestyle renderer. I hope you like it. Personally, I'm satisfied with the "technical illustration" appearance of the 3D renderings , but I'm not so happy with the 2D drafting work flow and results and am considering moving away from Blender to a 2D CAD program for those.

We are going to look at a carpentry model that would have been used to teach roofing layout principles to apprentices and young carpenters. Today, aspirants and apprentices spend two hours a day in night school where study of these kinds of models still figures heavily. The model is not to scale and is missing common rafters, but it has features that appear in almost all roofs in the French tradition: principal and half rafters, hip rafters that are backed to meet the slope of their adjoining surfaces, and purlins that are supported by the principal and hip rafters. As we discussed in my last post, the surfaces of the different rafters lie on imaginary solids that are similar to the overal roof shape. This isn't always the case, but it is a guiding principle in the layout. This model even has moving parts, to illustrate the drafting construction for finding the hip rafter section:

We begin the plan by laying out the shape of the roof and the principal rafters:

The "roof" is 64 x 102 centimeters, with a center height of 36.8 cm. The kingpost is 6.2cm x 6.8cm. Note that this shape is not similar to the rectangle of the whole roof. Therefore ridge lines don't meet the corners of the kingpost, giving its top a kind of funky truncated pyramid shape.

The principal rafters are 2.8cm wide. The top surface of the principal rafter lies 6.8 cm vertically below the roof surface. The bottom surface is 11.4745cm below the roof. There's no reason for that bizzare dimension, but I forget how I originally sized the rafter! In the drawing, the elevation view of the rafters is placed on top of a plan of half the roof. The cursive "N" stands for niveau or level. This indicates that that line is at the reference ground level of the whole plan.

Next, it is straightforward to construct the elevation view of the half rafters:

The roof line and half rafter surfaces must intersect the principal surfaces at the center line of the king post. So, the intersections in the principal elevation view can be "swung down" with a compass to construct the other elevation; the half-rafter is then constructed parallel to the roof surface. The rafter footprints are directly constructed from the elevation views and the known width of the rafters. Here's what we have in 3d:

It is easy to see the odd shape of the top of the king post. Also, notice that the half rafter meets the king post at a slightly lower height than the principal rafters; again, this is due to the difference between the king post section and the shape of the whole roof.

The rafters are fixed to the king post with mortise and tenon joints. The layout of these joints is an interesting subproblem. the top of each tenon is horizontal so the rafter can be slid into place, and the bottom must follow the bottom surface of its rafter. The tenons coming in from the four sides of the king post need to meet nicely in the middle.

I've sized the tenon for the half rafter at 0.8cm and given it a "V" shape that follows the ridge lines. The principal rafter tenons are sized proportionally to meet the half rafter tenons at the ridge lines. A portion of the principal rafter tenons is higher than the top of the half rafter tenons, so that is given a rectangular profile to avoid any voids in the middle of the king post:

An interesting result of constructing the tenons this way is that the interior of the mortise has the same shape as the roof:

We now turn our attention to the hip rafters. I'm going to give them the odd width of 5.037cm, for reasons that will soon become apparent. The hip is going to have a pentagonal shape: the top surfaces are aligned with the neighboring roof surfaces, the sides are plumb, and the bottom just touches the intersection of the upper surfaces of the principal and half rafters. The two plumb sides should have the same height, so in plan view the edges between the tops and sides intersect the ground plane on a line perpendicular to the axis of the hip. On the other hand, those edges should intersect the outer edges of the roof surface. How do we place a perpendicular line with the given constraints? The answer is a parallelogram construction:

Two lines of the given length i.e., the desired width of the rafter, are extended from the corner vertex, perpendicular to the ridge line in plan. We extend a line parallel to the left roof edge from the end of the near line, and a parallel to the near roof edge from the end of the far line. These lines' intersections with the roof edge give us the line we are looking for: each of lines with which we started form a parallelogram that includes this new line, so that line must have the same length as the original lines [Edited per Chris' comments]. Lets draw in the rest of the hip:

The inner edge of the hip rafter footprint is perpendicular to the axis of the rafter and meets the intersection of the upper rafter surfaces with the ground plane.

We can see the reason for the odd hip dimension now: the far edge of the hip meets the king post at the intersection of the left and far roof surfaces. We do this in order to give the hip enough width to have visible lip that sits against the near surface of the king post. Of course, this would give us problems if we constructed the far hip rafters, but we are not going to do that. This is not a scale model of a real roof; it only illustrates the layout on one side of a roof. We finish off the hip rafter's footprint by making its inner edge touch the "inner ridge line" formed by the top surfaces of the rafters.

It should be clear that I didn't start the layout of the hip by choosing a random dimension; I worked backwards from the king post and then measured the distance. However, the parallelogram construction for drawing the hip is a common technique, not just in French carpentry drawing but in other traditions as well, and it is important to be exposed to it.

After drawing the plan view of the hip rafter, we can derive the section of the rafter by using the technique in the previous blog entry for the triangular rafter. Draw an elevation view of the top of the hip rafter along its plan view, from point A to F, giving it the height of the king post at K. Choose a point X on the hip rafter and drop a line perpendicular to AK from there. This will be the point that is rotated down to the ground plane to begin the section construction, so we can choose the position of X to place Y, the rotated point, at a convenient location that is neither in the rafter footprint nor too far away in the middle of the drawing. Draw HZ, the intersection of the section plane with the ground. As explained in the last post, lines from Y to H and Z are the adjoining roof surfaces in the section drawing, so those give us the top of the rafter section.

The bottom of the section is found by locating the intersections of the bottom planes of the principal rafters with HZ. In the section view the lines showing these planes are parallel to the lines we already found for the roof surfaces. These new lines meet at U, giving us the bottom of the section.

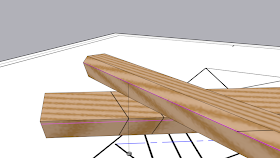
When we turn the hip rafter on its hinge, we see that its cross section does line up with our construction lines, and the bottom of the rafter passes through point U:

The next task is to find the final shape of the hip rafter where it meets the king post and mark the required cuts on a drawing so that a carpenter could cut it out, either by transferring measurements onto the timber or by creating the drawing on the ground and laying the timber on top of it. This implies that we need to make drawings of the hip rafter in its true proportions i.e., with the length, height and width square to our view. The elevation we constructed for finding the hip rafter section is a good start: it shows true length, and we can easily add the height of the hip rafter to the drawing. We will add another view of the rafter from below to show the width.

From our plan and 3d views of the model, we know that the hip rafter wraps around the king post in a kind of ell called a barbe or beard. We can treat the near and right sides of the king post as planes that cut through the hip rafter:

The portion of the hip rafter that needs to be cut away lies on the "inside" of both planes, in a Boolean intersection of the half spaces defined by the planes.

We will proceed by finding the intersections of those planes with the arrises of the hip rafter and then connecting them to form the lines of intersection of the hip rafter and king post surfaces. When these lines are marked out on the real timber, the carpenter can saw along them to establish the needed cuts. Obviously he shouldn't saw all the way through the wood, but it is reasonably straight-forward to saw along both sets of lines until their intersection, and no further.

In the plan view, extend the line of the near (red) king post surface to intersect all the edges of the hip rafter:

The left and right lines of the rafter in plan view represent upper and lower edges of the vertical surfaces of the rafter, so we will need to plot the intersections of each of them with the king post surfaces. There is only one top arris on the rafter, of course. We bring the intersections from the plan view up into the elevation view of the hip rafter, and from there into the rafter's bottom view. (Dashed lines in the rafter views show lines that are hidden by surfaces that are closer to the viewer; for various reasons the dashes may not show up well. I am hoping to fix that in future articles).

The procedure for the right (blue) king post plane is identical:

The joint between the hip rafter and the king post has been completely laid out. If the drawing were done at full scale on the workshop floor or construction site, the timber could be placed on top of e.g., the elevation drawing in order to transfer the intersections in the drawing to the timber. Marks on the right side of the timber can be transferred directly; the other marks need to be brought up the sides of the timber using a square.

Both the drafting and the timber marking methods are called rembarrement. This has nothing to do with rude behavior. The word refers to "pushing back" a point along a line from one context to another. When drafting the hip rafter, we moved the intersection points from plan view, where they were easy to find, into the much more useful elevation view. The carpenter moves marks from the floor up to edges using a square or plumb bob, perhaps making marks on intervening edges. These methods are still in use in carpentry drafting and layout. Rembarrement works well when using the straight and square timber that is easily available today. If we placed the hip rafter timber over its full-scale drawing and found that it was warped or out of square, we could probably fake it: use a corner and edge of the timber as a reference and mark out the lines. However, if the king post timber was out of square we would be kind of stuck. We don't use the real king post to lay out the hip rafter, but an abstraction of its surface planes that are perfectly flat and square. We could proceed by "fixing" those parts of the king post that do intersect with the rafter to be square. As I understand it, this is the "square rule" practice that started appearing in America in the early 18th century.

In Europe, carpenters had been dealing with out-of-square timbers in their carpentry drawings since the 13th century (at least). In the next installment we'll look at the layout method they used and how it is incorporated in the carpentry drawing.